You can use either P values or confidence intervals to determine whether your results are statistically significant. Confidence intervals describe the range within which a result for the whole population would occur for a specified proportion of times a survey or test was repeated among a sample of the population.

Effectively it measures how confident you are that the mean of your sample the sample mean is the same as the mean of the total population from which your sample was taken the population mean.
Statistical significance confidence interval. A confidence interval or confidence level is a range of values that have a given probability that the true value lies within it. Effectively it measures how confident you are that the mean of your sample the sample mean is the same as the mean of the total population from which your sample was taken the population mean. One way of measuring the degree of confidence in statistical results is to review the.
Confidence interval reported by researchers. Confidence intervals describe the range within which a result for the whole population would occur for a specified proportion of times a survey or test was repeated among a sample of the population. You can use either P values or confidence intervals to determine whether your results are statistically significant.
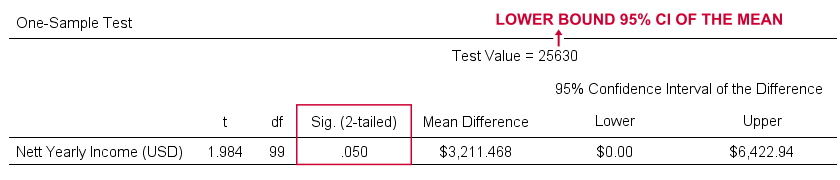
If a hypothesis test produces both these results will agree. If the confidence interval does not contain the null hypothesis value the results are statistically significant. The inference of statistical significance could have been made on the basis of the 95 confidence interval.
The 95 confidence interval for the population difference in mean birth weight was 711 to 1723 g. Because the 95 confidence interval straddled zero it can be inferred that the difference between treatment groups in mean birth weight was not significant at the 5 levelthat is. The 95 percent confidence interval for the first group mean can be calculated as.
919625 where 196 is the critical t-value. The confidence interval for the first group mean is thus 41139. Similarly for the second group the confidence interval for the mean is.
A critical value is the value of the test statistic which defines the upper and lower bounds of a confidence interval or which defines the threshold of statistical significance in a statistical test. It describes how far from the mean of the distribution you have to go to cover a certain amount of the total variation in the data ie. Understanding the confidence interval and statistical significance 0 I am struggling to understand confidence intervals and their relationships to a null hypothesis.
The basic definition of the confidence interval is. 1α where α is the statistical significance. Statistical Significance and Confidence Intervals If the two confidence intervals do not overlap we can conclude that there is a statistically significant difference in the two population values at the given level of confidence.
A 95 confidence interval CI of the mean is a range with an upper and lower number calculated from a sample. Because the true population mean is unknown this range describes possible values that the mean could be. If multiple samples were drawn from the same population and a 95 CI calculated for.
Confidence intervals provide all the information that a test of statistical significance provides and more. If at the 95 percent confidence level a confidence interval for an effect includes 0 then the test of significance would also indicate that the sample estimate was not. If the 95 confidence interval excludes unity then the test of the statistical hypotheses will be significant at the 5 level and the null hypothesis is rejected in favour of the alternative.
The 95 confidence interval for a ratio will never straddle zero the lower limit being above zero and the upper limit bounded by infinity b is false. In Statistics a confidence interval is a kind of interval calculation obtained from the observed data that holds the actual value of the unknown parameter. It is associated with the confidence level that quantifies the confidence level in which the interval estimates the deterministic parameter.
As described above the 95. Confidence interval is similar to the P value in that it can also. Be used to perform a dichotomous statistical hypothesis test at.
The 5 level of significance. It is 95 evident that average Age lies in the interval of 2652 years to 2221 years. The median of age is 225.
The age of the samples varies from 18 to 35 years. The mean height of the sampled responders is 164818 cm with standard deviation 6905 cm. The median of the heights of.
The correct interpretation of a 95 confidence interval is that we are 95 confident that the population parameter is between X and X What is the difference between a significance level and a P value. How do you know if a p-value is statistically significant. The level of statistical significance is often expressed as a p-value between 0.