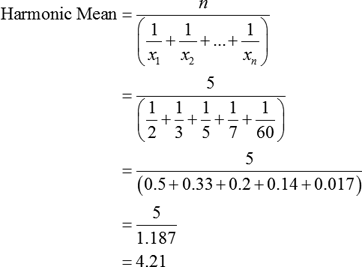
Statistics - Harmonic Mean Harmonic Mean is also a mathematical average but is limited in its application. Let x 1 x 2 x n be the n observations then the harmonic mean is defined as.

It is generally used to find average of variables that are expressed as a ratio.
Definition of harmonic mean in statistics. The Harmonic Mean HM is defined as the reciprocal of the average of the reciprocals of the data values. It is based on all the observations and it is rigidly defined. Harmonic mean gives less weightage to the large values and large.
Harmonic mean is a type of average that is calculated by dividing the number of values in a data series by the sum of the reciprocals 1x_i of each value in the data series. A harmonic mean is one of the three Pythagorean means the. Statistics - Harmonic Mean Harmonic Mean is also a mathematical average but is limited in its application.
It is generally used to find average of variables that are expressed as a ratio. Harmonic Mean in statistics is the reciprocal of the arithmetic mean of the values. It is based on all observations and is rigidly defined.
It is applied in the case of times and average rates. Relationship between Arithmetic mean Geometric Mean and Harmonic Mean. What Is a Harmonic Mean.
The harmonic mean is a type of numerical average. It is calculated by dividing the number of observations by the reciprocal of each number in the series. The Harmonic Mean HM is defined as the reciprocal of the arithmetic mean of the reciprocals of the observations.
Harmonic mean gives less weightage to the larger values and more weightage to the smaller values to balance the values properly. The harmonic mean involves taking the reciprocal of each number. The inverses are summed and the sum is divided into the number of data points which contributed to the sum.
Alternatively one could sum the inverses divide the sum by the number of data points and then find the inverse of the result. Harmonic mean is defined as the value obtained when the number of values in the data set is divided by the sum of its reciprocals. Moreover it is considered as one of the measures of central tendency.
Let x1x2xnbe the set of observations then the harmonic mean is given by where nrepresents the total number of observations. A simple way to define a harmonic mean is to call it the reciprocal of the arithmetic mean of the reciprocals of the observations. The most important criteria for it is that none of the observations should be zero.
A harmonic mean is used in averaging of ratios. The harmonic mean is the inverse of the arithmetic mean of the reciprocals of the observations of a set. It is a type of average generally used for numbers that represent a rate or ratio such as the precision and the recall in information retrieval and can be described as the reciprocal of the arithmetic mean of the reciprocals of the data.
Harmonic mean definition is - the reciprocal of the arithmetic mean of the reciprocals of a finite set of numbers. The harmonic mean has the following merits. It is rigidly defined.
It is based on all the observations of a series ie. It cannot be calculated ignoring any item of a series. It is capable of further algebraic treatment.
Harmonic Mean HM Harmonic Mean is defined as the reciprocal of the arithmetic mean of reciprocals of the observations. For Ungrouped data. Let x 1 x 2 x n be the n observations then the harmonic mean is defined as.
A man travels from Jaipur to Agra by a car and takes 4 hours to cover the whole distance. The three classical Pythagorean means are the arithmetic meanAM the geometric meanGM and the harmonic meanHM. The harmonic mean has the least value compared to the geometric and arithmetic.